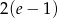Zadanie nr 1845619
Oblicz długość krzywej danej równaniami 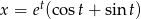 ,
, 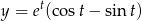 , gdzie
, gdzie ![t ∈ [0,1]](https://img.zadania.info/zad/1845619/HzadT2x.gif) .
.
Rozwiązanie
Korzystamy ze wzoru
![∫ b∘ ----------------- [x′(t)]2 + [y′(t)]2dt a](https://img.zadania.info/zad/1845619/HzadR0x.gif)
na długość krzywej o parametryzacji 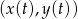 , gdzie
, gdzie ![t ∈ [a,b]](https://img.zadania.info/zad/1845619/HzadR2x.gif) .
.
Liczymy
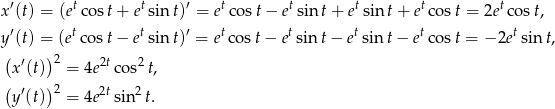
Mamy zatem
![∫ π ∘ ----------------- ∫ 1∘ ---------------------- 2 [x′(t)]2 + [y′(t)]2dt = 4e2tco s2t+ 4e2t sin 2tdt = 0 0 ∫ 1√ --- ∫ 1 t √ --[ t]π = 2 e2tdt = 2 edt = 2 e 20 = 2 (e − 1). 0 0](https://img.zadania.info/zad/1845619/HzadR4x.gif)
Odpowiedź: 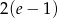
 Największy internetowy zbiór zadań z matematyki
Największy internetowy zbiór zadań z matematyki
Oblicz długość krzywej danej równaniami 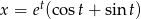 ,
, 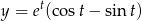 , gdzie
, gdzie ![t ∈ [0,1]](https://img.zadania.info/zad/1845619/HzadT2x.gif) .
.
Korzystamy ze wzoru
![∫ b∘ ----------------- [x′(t)]2 + [y′(t)]2dt a](https://img.zadania.info/zad/1845619/HzadR0x.gif)
na długość krzywej o parametryzacji 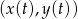 , gdzie
, gdzie ![t ∈ [a,b]](https://img.zadania.info/zad/1845619/HzadR2x.gif) .
.
Liczymy
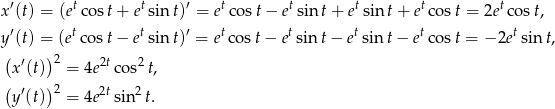
Mamy zatem
![∫ π ∘ ----------------- ∫ 1∘ ---------------------- 2 [x′(t)]2 + [y′(t)]2dt = 4e2tco s2t+ 4e2t sin 2tdt = 0 0 ∫ 1√ --- ∫ 1 t √ --[ t]π = 2 e2tdt = 2 edt = 2 e 20 = 2 (e − 1). 0 0](https://img.zadania.info/zad/1845619/HzadR4x.gif)
Odpowiedź: