Zadanie nr 6387468
Oblicz całkę 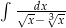 .
.
Rozwiązanie
Sposób I
Ponieważ

podstawiamy 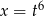 .
.
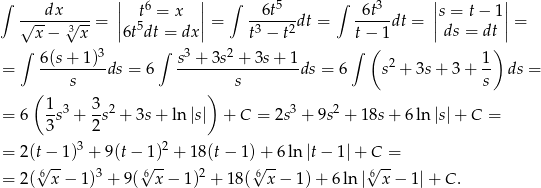
Sposób II
Liczymy podstawiając 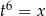 .
.
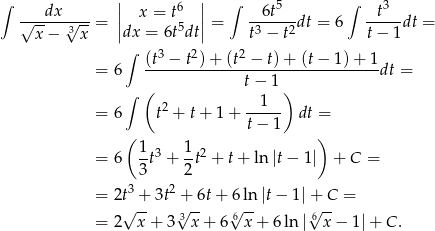
Odpowiedź: 
 Największy internetowy zbiór zadań z matematyki
Największy internetowy zbiór zadań z matematyki
Oblicz całkę 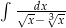 .
.
Sposób I
Ponieważ

podstawiamy 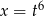 .
.
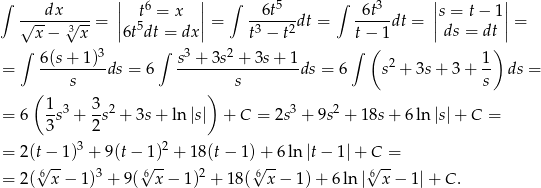
Sposób II
Liczymy podstawiając 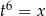 .
.
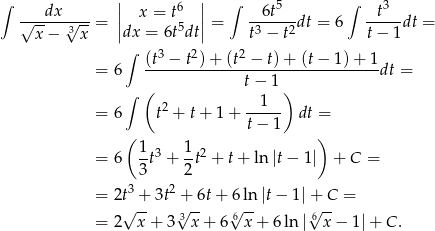
Odpowiedź: 
